The Astrolabe Project

Two videos I ran across on YouTube:

The first is a good, quick introduction to the tool LINK.

The second is a very nice breakdown of a practical use of the astrolabe LINK.

The next step, and the last scale to be added to the back of my astrolabe, is the unequal hour scale.

In the middle ages, there where several timekeeping systems in use, as opposed to the single one we use now. This next scale was designed to convert between two of these systems: The Equal Hour and Unequal Hour systems.

The Equal Hour system is the one you are familiar with; 24 hours of 60 minutes each. The Unequal Hour system is different: The day (sunrise to sunset) and the night (sunset to sunrise) are each    divided into 12 hours, regardless of the time of year. Therefore in Summer an hour of daylight is longer than an hour of    darkness, and in Winter the reverse is true. The sun rises at the start of the first hour of the day and sets at the end of the 12th hour of the day, which is also the start of the first hour of the night; and local noon marks the end of the 6th hour.

Further discussion and examples can be found in my class handout.

The completed scale looks like this:

Depending on the astrolabe the scale may be on either or both sides, as it is symmetrical.

Drawing the unequal hour arcs is relatively straightforward once you understand the trick. The following is adapted from Stoeffler and Morrison (see recommended reading ).

First, draw an arc just inside the innermost ring; centered on the center of the astrolabe, and forming a half-circle above the shadow squares.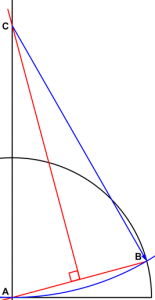

Next divide the arc into twelve equal parts. Stoeffler suggests that you use the outer elevation scale and make a mark every 15 degrees.

Now look at the figure above. Note that because the scale is symmetrical, the center of each arc will lie somewhere on the vertical center-line. Note also that each arc touches two points: The astrolabe central pivot, and one of the marks just made, therefore these two points must be an equal distance from the arc center.

This means that you can find the center of each arc by raising a perpendicular bisector to the line between the two points and marking where it crosses the center line (see Drafting the Astrolabe: 2. Methods of geometric construction).

Step1: Draw a light construction line from Point A (the astrolabe center) to Point B (any one of the twelve fifteen-degree hour lines).

Step 2: Erect a perpendicular bisector from line AB to the astrolabe mid-line and mark it Point C.

Step 3: Place the point of your compass at Point C and draw an arc from Point A to Point B (mirrored on both sides if that is your wish).

Step 4: Repeat until all the arcs are drawn. Erase construction lines and label the arcs as shown above.

The Missing Manual has gone missing!

As some of you might have noticed, the Joomla site for Astrolabe: The Missing Manual is gone. Like the original wiki it replaced, it was not meeting my needs, so I nuked it.  Do not despair! The manual still exists, and is still being added to regularly (more regularly than this blog lately). I replaced an overly complicated site with a simple PDF. The link is on the right as “Astrolabe Manual”… I will update this file as I add to the manual, and will provide version information inside the front cover.

Plans and instructions for making a plastic astrolabe

I stumbled across this today at Thingverse: A plastic working astrolabe. The creator made it back in June 2010, and didn’t stumble across my site until afterword. Here’s hoping that someone will be inspired to make more…

There’s an app for that

Or there will be soon. I was contacted by a developer who is working on an iPad app that simulates various medieval astronomical devices. He will be using files made with the Astrolabe Generator. I’ll post information here when it becomes available.

I thought I posted this two weeks ago… Work and life have gotten busier than I realized it seems.

Anyway, here is the completed shadow square section. Next up will be the unequal hours scale and then the back will be done.

Getting the zodiac and calendar rings done was probably the worse, most finicky part of this project; the rest of the tasks remaining might require careful measurement, and attention to detail, but orders of magnitude less work than the last section.
The next task is to finish off the rear of the astrolabe. Two scales remain to be drawn, the Unequal hours and the shadow squares.

Drafting the shadow squares is straight-forward: Two square boxes in the bottom half of the astrolabe back, each side divided into a set number of sections. The only trick bit will be finding the correct size for the boxes. I want the shadow squares to be as big as possible, as larger means more accurate to draw and to use, but they also need to fit into the remaining space inside the calendar ring. Because the calendar ring is offset, I have to take that offset into account

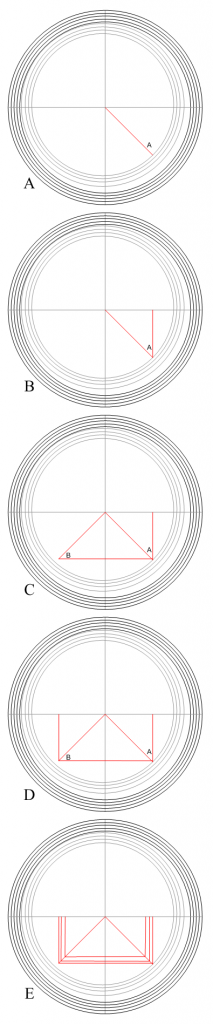
The largest dimension of the shadow square is its 45 degree diagonal, so if I draw a line at 45 degrees from the center to the right bottom side of the astrolabe, stopping at the inside edge of the calendar ring, I can use that point to erect the rest of the square.

Step 1: Draw the 45 degree diagonal from the center down and right to where it touches the inside of the calendar ring on the bottom right side of the astrolabe back. Mentally label this point “A” (See Figure A).

Step 2: Erect a vertical line from point A to the horizontal center line of the astrolabe (see Figure B).

Step 3: Erect a line parallel to the horizontal center line of the astrolabe, running from point A to the left side of the astrolabe (see Figure C).

Step 4: Draw the 45 degree diagonal from the center down and left to where it touches the line drawn in the previous step. Mentally label this point “B” (see Figure C).

Step 5: Erect a vertical line from point B to the horizontal center line of the astrolabe as you did in step 2 (see Figure D).

Step 6: Draw a scale line inside the box you just drew (see Figure E).

Step 7: Divide and label the Shadow Squares.

The inking of the calendar ring is done. The result is not as neat as I might wish, But it is workable. The next step will be much easier: Drawing the shadow squares.

While I’m working on this project, I’m also researching the tools and techniques used by the instrument makers who made these astrolabes. The two period manuals I am working from assume a certain shared level of knowledge, and do not mention tools or engraving techniques, except in passing.

I am finding the art of the period a better resource; in particular, I found a very nice portrait by Hans Holbein the Younger:

Nicholas Kratzer by Hans Holbein the Younger

(Note: WikiMedia is listing this image as Public Domain, if anyone claims the rights to the photograph, please contact me at the email to the right and we can discuss it)

The original image can be found here.

The subject of the portrait is shown with several tools and a partially completed sundial compendium similar to those described in Hartmann. The tools for designing and laying out the lines of a sundial are the same ones that would be needed in designing and laying out an astrolabe.

Detail of tools

Detail of tools

Identifiable in the portrait are two straight-edges (one on the table, one hanging), two scribing compasses (on the table and hanging), a set of dividers (by the hammer on the right), a scriber (in front, right of center), some scissors and some other tools whose use is not immediately evident.

My friend Miguel, of Spanish Peacock was nice enough to make me a very nice straight-edge out of hardwood, based on the one in the painting:

Tools for Astrolabe Construction

A bit later, I stumbled across a two pairs of scribing dividers at a Harbor Freight sale.

I’m currently looking into buying a good scriber, and I will be ready to start playing with brass. I have neither the time nor skill to build a complete astrolabe from scratch, but I’m thinking a brass version of one of the climate plates might be instructive to attempt.